

RCC Structures Design - Engineering
Q1: A prestressed rectangular beam which carries two concentrated loads W at L/3 from either end, is provided with a bent tendon with tension P such that central one-third portion of the tendon remains parallel to the longitudinal axis, the maximum dip h isA
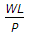
B
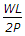
C
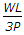
D
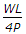
E
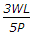
ANS:C -
In a prestressed rectangular beam carrying two concentrated loads W at 33L from either end and provided with a bent tendon with tension P such that the central one-third portion of the tendon remains parallel to the longitudinal axis, the maximum dip ℎh of the tendon can be calculated using the principles of prestressed concrete. The maximum dip ℎh occurs at the midspan of the beam, where the bending moment due to the applied loads and the prestressing force is maximum. The tendon profile is typically parabolic under uniform load, and the maximum dip occurs at the midspan where the shear force is zero. The formula to calculate the maximum dip ℎh of the tendon in a prestressed rectangular beam is given by: h=8EIPL2 Where:
|


For help Students Orientation
Mcqs Questions
One stop destination for examination, preparation, recruitment, and more. Specially designed online test to solve all your preparation worries. Go wherever you want to and practice whenever you want, using the online test platform.

