Download Aptitude Crack
img not found img not found

Permutation and Combination - General Aptitude

Q1:

In a group of 6 boys and 4 girls, four children are to be selected. In how many different ways can they be selected such that at least one boy should be there?

A 159

B 194

C 205

D 209

E None of these

ANS:D - 209

We may have (1 boy and 3 girls) or (2 boys and 2 girls) or (3 boys and 1 girl) or (4 boys).

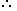 Required number
of ways		 = (6C1 x 4C3) + (6C2 x 4C2) + (6C3 x 4C1) + (6C4)
  = (6C1 x 4C1) + (6C2 x 4C2) + (6C3 x 4C1) + (6C2)
 
= (6 x 4) + 6 x 5 x 4 x 3 + 6 x 5 x 4 x 4 + 6 x 5
2 x 1 2 x 1 3 x 2 x 1 2 x 1
  = (24 + 90 + 80 + 15)
  = 209.