

Waste Water Engineering - Engineering
Q1: The settling velocity of a spherical particle of diameter less than 0.1 mm as per Stock's law, isA
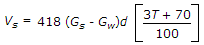
B

C

D

ANS:B -
Stocks's law describes the settling velocity of small spherical particles in a fluid medium. It states that the settling velocity (��Vs) of a small spherical particle is directly proportional to the square of its diameter (�d). Mathematically, Stocks's law can be expressed as: ��=��2Vs=kd2 Where:
|


For help Students Orientation
Mcqs Questions
One stop destination for examination, preparation, recruitment, and more. Specially designed online test to solve all your preparation worries. Go wherever you want to and practice whenever you want, using the online test platform.

